3.9: Useful techniques - demand and supply equations

Last updated
Save as PDF

Page ID: 108373

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $ $ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $$\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$ $\newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\kernel}{\mathrm{null}\,}$ $ \newcommand{\range}{\mathrm{range}\,}$ $ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$ $ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$ $ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$ $ \newcommand{\Span}{\mathrm{span}}$$\newcommand{\AA}{\unicode[.8,0]{x212B}}$

The supply and demand functions, or equations, underlying Table 3.1 and Figure 3.2 can be written in their mathematical form:

A straight line is represented completely by the intercept and slope. In particular, if the variable P is on the vertical axis and Q on the horizontal axis, the straight-line equation relating P and Q is defined by P=a+bQ. Where the line is negatively sloped, as in the demand equation, the parameter b must take a negative value. By observing either the data in Table 3.1 or Figure 3.2 it is clear that the vertical intercept, a, takes a value of $10. The vertical intercept corresponds to a zero-value for the Q variable. Next we can see from Figure 3.2 that the slope (given by the rise over the run) is 10/10 and hence has a value of –1. Accordingly the demand equation takes the form P=10–Q.

On the supply side the price-axis intercept, from either the figure or the table, is clearly 1. The slope is one half, because a two-unit change in quantity is associated with a one-unit change in price. This is a positive relationship obviously so the supply curve can be written as P=1+(1/2)Q.

Where the supply and demand curves intersect is the market equilibrium; that is, the price-quantity combination is the same for both supply and demand where the supply curve takes on the same values as the demand curve. This unique price-quantity combination is obtained by equating the two curves: If Demand=Supply, then

10–Q=1+(1/2)Q.

Gathering the terms involving Q to one side and the numerical terms to the other side of the equation results in 9=1.5Q. This implies that the equilibrium quantity must be 6 units. And this quantity must trade at a price of $4. That is, when the price is $4 both the quantity demanded and the quantity supplied take a value of 6 units.

Modelling market interventions using equations

To illustrate the impact of market interventions examined in Section 3.7 on our numerical market model for natural gas, suppose that the government imposes a minimum price of $6 – above the equilibrium price obviously. We can easily determine the quantity supplied and demanded at such a price. Given the supply equation

P=1+(1/2)Q,

it follows that at P=6 the quantity supplied is 10. This follows by solving the relationship 6=1+(1/2)Q for the value of Q. Accordingly, suppliers would like to supply 10 units at this price.

Correspondingly on the demand side, given the demand curve

P=10–Q,

with a price given by 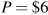, it must be the case that Q=4. So buyers would like to buy 4 units at that price: There is excess supply. But we know that the short side of the market will win out, and so the actual amount traded at this restricted price will be 4 units.