9.1: The Production Function

Last updated
Save as PDF

Page ID: 3484

Boundless
Boundless

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$ \newcommand{\id}{\mathrm{id}}$ $ \newcommand{\Span}{\mathrm{span}}$

( \newcommand{\kernel}{\mathrm{null}\,}\) $ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$ $ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$ $ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\id}{\mathrm{id}}$

$ \newcommand{\Span}{\mathrm{span}}$

$ \newcommand{\kernel}{\mathrm{null}\,}$

$ \newcommand{\range}{\mathrm{range}\,}$

$ \newcommand{\RealPart}{\mathrm{Re}}$

$ \newcommand{\ImaginaryPart}{\mathrm{Im}}$

$ \newcommand{\Argument}{\mathrm{Arg}}$

$ \newcommand{\norm}[1]{\| #1 \|}$

$ \newcommand{\inner}[2]{\langle #1, #2 \rangle}$

$ \newcommand{\Span}{\mathrm{span}}$ $ \newcommand{\AA}{\unicode[.8,0]{x212B}}$

$ \newcommand{\vectorA}[1]{\vec{#1}} % arrow$

$ \newcommand{\vectorAt}[1]{\vec{\text{#1}}} % arrow$

$ \newcommand{\vectorB}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vectorC}[1]{\textbf{#1}} $

$ \newcommand{\vectorD}[1]{\overrightarrow{#1}} $

$ \newcommand{\vectorDt}[1]{\overrightarrow{\text{#1}}} $

$ \newcommand{\vectE}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash{\mathbf {#1}}}} $

$ \newcommand{\vecs}[1]{\overset { \scriptstyle \rightharpoonup} {\mathbf{#1}} } $

$ \newcommand{\vecd}[1]{\overset{-\!-\!\rightharpoonup}{\vphantom{a}\smash {#1}}} $

$\newcommand{\avec}{\mathbf a}$ $\newcommand{\bvec}{\mathbf b}$ $\newcommand{\cvec}{\mathbf c}$ $\newcommand{\dvec}{\mathbf d}$ $\newcommand{\dtil}{\widetilde{\mathbf d}}$ $\newcommand{\evec}{\mathbf e}$ $\newcommand{\fvec}{\mathbf f}$ $\newcommand{\nvec}{\mathbf n}$ $\newcommand{\pvec}{\mathbf p}$ $\newcommand{\qvec}{\mathbf q}$ $\newcommand{\svec}{\mathbf s}$ $\newcommand{\tvec}{\mathbf t}$ $\newcommand{\uvec}{\mathbf u}$ $\newcommand{\vvec}{\mathbf v}$ $\newcommand{\wvec}{\mathbf w}$ $\newcommand{\xvec}{\mathbf x}$ $\newcommand{\yvec}{\mathbf y}$ $\newcommand{\zvec}{\mathbf z}$ $\newcommand{\rvec}{\mathbf r}$ $\newcommand{\mvec}{\mathbf m}$ $\newcommand{\zerovec}{\mathbf 0}$ $\newcommand{\onevec}{\mathbf 1}$ $\newcommand{\real}{\mathbb R}$ $\newcommand{\twovec}[2]{\left[\begin{array}{r}#1 \\ #2 \end{array}\right]}$ $\newcommand{\ctwovec}[2]{\left[\begin{array}{c}#1 \\ #2 \end{array}\right]}$ $\newcommand{\threevec}[3]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\cthreevec}[3]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \end{array}\right]}$ $\newcommand{\fourvec}[4]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\cfourvec}[4]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \end{array}\right]}$ $\newcommand{\fivevec}[5]{\left[\begin{array}{r}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\cfivevec}[5]{\left[\begin{array}{c}#1 \\ #2 \\ #3 \\ #4 \\ #5 \\ \end{array}\right]}$ $\newcommand{\mattwo}[4]{\left[\begin{array}{rr}#1 \amp #2 \\ #3 \amp #4 \\ \end{array}\right]}$ $\newcommand{\laspan}[1]{\text{Span}\{#1\}}$ $\newcommand{\bcal}{\cal B}$ $\newcommand{\ccal}{\cal C}$ $\newcommand{\scal}{\cal S}$ $\newcommand{\wcal}{\cal W}$ $\newcommand{\ecal}{\cal E}$ $\newcommand{\coords}[2]{\left\{#1\right\}_{#2}}$ $\newcommand{\gray}[1]{\color{gray}{#1}}$ $\newcommand{\lgray}[1]{\color{lightgray}{#1}}$ $\newcommand{\rank}{\operatorname{rank}}$ $\newcommand{\row}{\text{Row}}$ $\newcommand{\col}{\text{Col}}$ $\renewcommand{\row}{\text{Row}}$ $\newcommand{\nul}{\text{Nul}}$ $\newcommand{\var}{\text{Var}}$ $\newcommand{\corr}{\text{corr}}$ $\newcommand{\len}[1]{\left|#1\right|}$ $\newcommand{\bbar}{\overline{\bvec}}$ $\newcommand{\bhat}{\widehat{\bvec}}$ $\newcommand{\bperp}{\bvec^\perp}$ $\newcommand{\xhat}{\widehat{\xvec}}$ $\newcommand{\vhat}{\widehat{\vvec}}$ $\newcommand{\uhat}{\widehat{\uvec}}$ $\newcommand{\what}{\widehat{\wvec}}$ $\newcommand{\Sighat}{\widehat{\Sigma}}$ $\newcommand{\lt}{<}$ $\newcommand{\gt}{>}$ $\newcommand{\amp}{&}$ $\definecolor{fillinmathshade}{gray}{0.9}$

Defining the Production Function

The production function relates the maximum amount of output that can be obtained from a given number of inputs.

learning objectives

Define the production function

In economics, a production function relates physical output of a production process to physical inputs or factors of production. It is a mathematical function that relates the maximum amount of output that can be obtained from a given number of inputs – generally capital and labor. The production function, therefore, describes a boundary or frontier representing the limit of output obtainable from each feasible combination of inputs.

Firms use the production function to determine how much output they should produce given the price of a good, and what combination of inputs they should use to produce given the price of capital and labor. When firms are deciding how much to produce they typically find that at high levels of production, their marginal costs begin increasing. This is also known as diminishing returns to scale – increasing the quantity of inputs creates a less-than-proportional increase in the quantity of output. If it weren’t for diminishing returns to scale, supply could expand without limits without increasing the price of a good.

Factory Production: Manufacturing companies use their production function to determine the optimal combination of labor and capital to produce a certain amount of output.

Increasing marginal costs can be identified using the production function. If a firm has a production function $Q=F(K,L)$ (that is, the quantity of output (Q) is some function of capital (K) and labor (L)), then if $2Q<F(2K,2L)$, the production function has increasing marginal costs and diminishing returns to scale. Similarly, if $2Q>F(2K,2L)$, there are increasing returns to scale, and if $2Q=F(2K,2L)$, there are constant returns to scale.

Examples of Common Production Functions

One very simple example of a production function might be $Q=K+L$, where Q is the quantity of output, K is the amount of capital, and L is the amount of labor used in production. This production function says that a firm can produce one unit of output for every unit of capital or labor it employs. From this production function we can see that this industry has constant returns to scale – that is, the amount of output will increase proportionally to any increase in the amount of inputs.

Another common production function is the Cobb-Douglas production function. One example of this type of function is $Q=K^{0.5}L^{0.5}$. This describes a firm that requires the least total number of inputs when the combination of inputs is relatively equal. For example, the firm could produce 25 units of output by using 25 units of capital and 25 of labor, or it could produce the same 25 units of output with 125 units of labor and only one unit of capital.

Finally, the Leontief production function applies to situations in which inputs must be used in fixed proportions; starting from those proportions, if usage of one input is increased without another being increased, output will not change. This production function is given by $Q=Min(K,L)$. For example, a firm with five employees will produce five units of output as long as it has at least five units of capital.

The Law of Diminishing Returns

The law of diminishing returns states that adding more of one factor of production will at some point yield lower per-unit returns.

Learning Objectives

Explain the Law of Diminishing Returns

In economics, diminishing returns (also called diminishing marginal returns) is the decrease in the marginal output of a production process as the amount of a single factor of production is increased, while the amounts of all other factors of production stay constant. The law of diminishing returns states that in all productive processes, adding more of one factor of production, while holding all others constant (“ceteris paribus”), will at some point yield lower per-unit returns. The law of diminishing returns does not imply that adding more of a factor will decrease the total production, a condition known as negative returns, though in fact this is common.

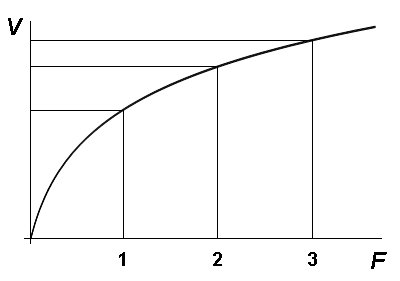
Diminishing Returns: As a factor of production (F) increases, the resulting gain in the volume of output (V) gets smaller and smaller.

For example, the use of fertilizer improves crop production on farms and in gardens; but at some point, adding more and more fertilizer improves the yield less per unit of fertilizer, and excessive quantities can even reduce the yield. A common sort of example is adding more workers to a job, such as assembling a car on a factory floor. At some point, adding more workers causes problems such as workers getting in each other’s way or frequently finding themselves waiting for access to a part. In all of these processes, producing one more unit of output will eventually cost increasingly more, due to inputs being used less and less effectively.

This increase in the marginal cost of output as production increases can be graphed as the marginal cost curve, with quantity of output on the x axis and marginal cost on the y axis. For many firms, the marginal cost curve will initially be downward sloping, representing added efficiency as production increases. If the law of diminishing returns holds, however, the marginal cost curve will eventually slope upward and continue to rise, representing the higher and higher marginal costs associated with additional output.

The Law of Diminishing Returns and Average Cost

The average total cost of production is the total cost of producing all output divided by the number of units produced. For example, if the car factory can produce 20 cars at a total cost of $200,000, the average cost of production is $10,000. Average total cost is interpreted as the the cost of a typical unit of production. So in our example each of the 20 cars produced had a typical cost per unit of $10,000. Average total cost can also be graphed with quantity of output on the x axis and average cost on the y-axis.

What will this average total cost curve look like? In the short run, a firm has a set amount of capital and can only increase or decrease production by hiring more or less labor. The fixed costs of capital are high, but the variable costs of labor are low, so costs increase more slowly than output as production increases. As long as the marginal cost of production is lower than the average total cost of production, the average cost is decreasing. However, as marginal costs increase due to the law of diminishing returns, the marginal cost of production will eventually be higher than the average total cost and the average cost will begin to increase. The short run average total cost curve (SRAC) will therefore be U-shaped for most firms.

Cost Curves in the Short Run: Both marginal cost and average cost are U-shaped due to first increasing, and then diminishing, returns. Average cost begins to increase where it intersects the marginal cost curve.

The long-run average cost curve (LRAC) depicts the cost per unit of output in the long run—that is, when all productive inputs’ usage levels can be varied. The typical LRAC curve is also U-shaped but for different reasons: it reflects increasing returns to scale where negatively-sloped, constant returns to scale where horizontal, and decreasing returns (due to increases in factor prices) where positively sloped.

Inputs and Outputs of the Function

In the basic production function, inputs are typically capital and labor and output is whatever good the firm produces.

Learning Objectives

Describe the inputs and outputs in a generalized production function

A production function relates the input of factors of production to the output of goods. In the basic production function inputs are typically capital and labor, though more expansive and complex production functions may include other variables such as land or natural resources. Output may be any consumer good produced by a firm. Cars, clothing, sandwiches, and toys are all examples of output.

Capital refers to the material objects necessary for production. Machinery, factory space, and tools are all types of capital. In the short run, economists assume that the level of capital is fixed – firms can’t sell machinery the moment it’s no longer needed, nor can they build a new factory and start producing goods there immediately. When looking at the production function in the short run, therefore, capital will be a constant rather than a variable. Although in reality a firm may own the capital that it uses, economists typically refer to the ongoing cost of employing capital as the rental rate because the opportunity cost of employing capital is the income that a firm could receive by renting it out. Thus, the price of capital is the rental rate.

Capital Goods: Capital equipment, like these motor graders, can vary in the long run but are fixed in the short run.

Labor refers to the human work that goes into production. Typically economists assume that labor is a variable factor of production; it can be increased or decreased in the short run in order to produce more or less output. The price of labor is the prevailing wage rate, since wages are the cost of hiring an additional unit of capital.

The marginal product of an input is the amount of output that is gained by using one additional unit of that input. It can be found by taking the derivative of the production function in terms of the relevant input. For example, if the production function is Q=3K+2L (where K represents units of capital and L represents units of labor), then the marginal product of capital is simply three; every additional unit of capital will produce an additional three units of output. Inputs are typically subject to the law of diminishing returns: as the amount of one factor of production increases, after a certain point the marginal product of that factor declines.

Key Points

The production function describes a boundary or frontier representing the limit of output obtainable from each feasible combination of inputs.
Firms use the production function to determine how much output they should produce given the price of a good, and what combination of inputs they should use to produce given the price of capital and labor.
The production function also gives information about increasing or decreasing returns to scale and the marginal products of labor and capital.
One consequence of the law of diminishing returns is that producing one more unit of output will eventually cost increasingly more, due to inputs being used less and less effectively.
The marginal cost curve will initially be downward sloping, representing added efficiency as production increases. If the law of diminishing returns holds, however, the marginal cost curve will eventually slope upward and continue to rise.
The SRAC is typically U-shaped with its minimum at the point where it intersect the marginal cost curve. This is caused by the first increasing, and then decreasing, marginal returns to labor.
The typical LRAC curve is also U-shaped, reflecting increasing returns of scale where negatively-sloped, constant returns to scale where horizontal and decreasing returns where positively sloped.
Capital refers to the material objects necessary for production. In the short run, economists assume that the level of capital is fixed.
Labor refers to the human work that goes into production. Typically economists assume that labor is a variable factor of production.
The marginal product of an input is the amount of output that is gained by using one additional unit of that input. It can be found by taking the derivative of the production function in terms of the relevant input.

Key Terms

Production function: Relates physical output of a production process to physical inputs or factors of production.
marginal cost: The increase in cost that accompanies a unit increase in output; the partial derivative of the cost function with respect to output. Additional cost associated with producing one more unit of output.
output: Production; quantity produced, created, or completed.
returns to scale: A term referring to changes in output resulting from a proportional change in all inputs (where all inputs increase by a constant factor).
rental rate: The price of capital.
marginal product: The extra output that can be produced by using one more unit of the input.
capital: Already-produced durable goods available for use as a factor of production, such as steam shovels (equipment) and office buildings (structures).

LICENSES AND ATTRIBUTIONS

CC LICENSED CONTENT, SPECIFIC ATTRIBUTION

marginal cost. Provided by: Wiktionary. Located at: en.wiktionary.org/wiki/marginal_cost. License: CC BY-SA: Attribution-ShareAlike
Production function. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/Production_function. License: CC BY-SA: Attribution-ShareAlike
output. Provided by: Wiktionary. Located at: en.wiktionary.org/wiki/output. License: CC BY-SA: Attribution-ShareAlike
Production function. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/Production%20function. License: CC BY-SA: Attribution-ShareAlike
Wolfsburg VW-Werk. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/File:Wo...rg_VW-Werk.jpg. License: CC BY: Attribution
marginal cost. Provided by: Wiktionary. Located at: en.wiktionary.org/wiki/marginal_cost. License: CC BY-SA: Attribution-ShareAlike
Diminishing returns. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/Diminishing_returns. License: CC BY-SA: Attribution-ShareAlike
Cost curve. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/Cost_cu...un_cost_curves. License: CC BY-SA: Attribution-ShareAlike
returns to scale. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/returns%20to%20scale. License: CC BY-SA: Attribution-ShareAlike
Wolfsburg VW-Werk. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/File:Wo...rg_VW-Werk.jpg. License: CC BY: Attribution
Diminishing return. Provided by: Wikimedia. Located at: commons.wikimedia.org/wiki/Fi...ing_return.jpg. License: CC BY-SA: Attribution-ShareAlike
Profit max marginal small. Provided by: Wikimedia. Located at: commons.wikimedia.org/wiki/Fi...inal_small.svg. License: CC BY-SA: Attribution-ShareAlike
IB Economics/Microeconomics/Theory of the Firm (HL). Provided by: Wikibooks. Located at: en.wikibooks.org/wiki/IB_Econ..._the_Firm_(HL). License: CC BY-SA: Attribution-ShareAlike
Production theory. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/Production_theory. License: CC BY-SA: Attribution-ShareAlike
Boundless. Provided by: Boundless Learning. Located at: www.boundless.com//economics/...on/rental-rate. License: CC BY-SA: Attribution-ShareAlike
marginal product. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/marginal%20product. License: CC BY-SA: Attribution-ShareAlike
capital. Provided by: Wiktionary. Located at: en.wiktionary.org/wiki/capital. License: CC BY-SA: Attribution-ShareAlike
Wolfsburg VW-Werk. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/File:Wo...rg_VW-Werk.jpg. License: CC BY: Attribution
Diminishing return. Provided by: Wikimedia. Located at: commons.wikimedia.org/wiki/Fi...ing_return.jpg. License: CC BY-SA: Attribution-ShareAlike
Profit max marginal small. Provided by: Wikimedia. Located at: commons.wikimedia.org/wiki/Fi...inal_small.svg. License: CC BY-SA: Attribution-ShareAlike
FM CN 668080. Provided by: Wikipedia. Located at: en.Wikipedia.org/wiki/File:FM_CN_668080.jpg. License: CC BY-SA: Attribution-ShareAlike

Search

Text Color

Text Size

Margin Size

Font Type

learning objectives

Learning Objectives

Learning Objectives