The key idea is that every consumer has a set of likes and dislikes, desires, and tastes, called preferences. Consumer preferences enable them to compare any two combinations or bundles of goods and services in terms of better/worse or the same. The result of such a comparison has two outcomes:
Strictly preferred: the consumer likes one bundle better than the other.
Indifferent: the consumer is equally satisfied with the two bundles.
In terms of algebra, you can think of strictly preferred as greater than (\(>\)), indifferent as equal (=).
Since the consumer can compare any two bundles, then by repeated comparison of different bundles the consumer can rank all possible combinations from best to worst (in the consumer’s opinion).
Three Axioms
Three fundamental assumptions are made about preferences to ensure internal consistency:
Completeness: the consumer can compare any bundles and render a preferred or indifferent judgment.
Reflexivity: this identity condition says that the consumer is indifferent when comparing a bundle to itself.
Transitivity: this condition defines an orderly relation among bundles so that if bundle A is preferred to bundle B and bundle B is preferred to bundle C then bundle A must be preferred to bundle C.
Completeness and reflexivity are easily accepted. Transitivity, on the other hand, is controversial. As a matter of pure logic, we would expect that a consumer would make consistent comparisons. In practice, however, consumers may make intransitive, or inconsistent, choices.
An example of intransitivity: You claim to like Coke better than Pepsi, Pepsi better than RC, and RC better than Coke. The last claim is inconsistent with the first two. If Coke beats Pepsi and Pepsi beats RC, then Coke must really beat RC!
In mathematics, numbers are transitive with respect to the comparison operators greater than, less than, or equal to. Because 12 is greater than 8 and 8 is greater than 3, clearly 12 is greater than 3.
Sports results, however, are not like math. Outcomes of games can easily yield intransitive results. Michigan might beat Indiana and in its next game Indiana could defeat Iowa, but few people would claim that the two outcomes would guarantee that Michigan will win when it plays Iowa.
When we assume that preferences are transitive, it means that the consumer can rank bundles without any contradictions. It also means that we are able to determine the consumer’s choice between two bundles based on answers to previous comparisons.
Displaying Preferences via Indifference Curves
The consumer’s preferences can be revealed by having her choose between bundles. We can describe a consumer’s preferences with an indifference map, which is made up of indifference curves.
A single indifference curve is the set of combinations that give equal satisfaction. If two points lie on the same indifference curve, this means that the consumer sees these two bundles as tied – neither one is better nor worse than the other.
A single indifference curve and an entire indifference map can be generated by having the consumer choose between alternative bundles of goods. We can demonstrate how this works with a concrete example.
STEP Open the Excel workbook Preferences.xls, read the Intro sheet, and then go to the Reveal sheet to see how preferences can be mapped and the indifference curve revealed.
STEP Begin by clicking the button. For bundle B, enter 4, then a comma (,), then a 3, then click OK.
We are using the coordinate pair notation so 4,3 identifies a combination that has 4 units of the good on the x axis and 3 units of the good on the y axis.
The sheet records the bundles that are being compared in columns A and B and the outcome in column C. The choices are being made by a virtual consumer whose unknown preferences are in the computer. By asking the virtual consumer to make a series of comparisons, we can reveal the hidden preferences in the form of an indifference curve and indifference map.
Notice that Excel plots the point 4,3 on the chart. The green square means the consumer chose bundle B. This means that 3,3 and 4,3 are not on the same indifference curve.
STEP Click the button again. Offer the consumer a choice between 3,3 and 2,3.
This time the consumer chose bundle A and a red triangle was placed on the chart, meaning that the point 3,3 is strictly preferred to the point 2,3.
These two choices illustrate insatiability. This means that the consumer cannot be sated (or filled up) so more is always better. The combination 4,3 is preferred to 3,3, which is preferred to 2,3 because good \(x_{2}\) is held constant at 3 and this consumer is insatiable, preferring more of good \(x_{1}\) to less.
To reveal the indifference curve of this consumer, we must offer tougher choices, where we give more of one good and less of the other.
STEP Click the button again. This time offer the consumer a choice between 3,3 and 4,2.
The consumer decided that 3,3 is better. This reveals important information about the consumer’s preferences. At 3,3, the consumer likes one more unit of \(x_{1}\) less than the loss of one unit of \(x_{2}\).
STEP Click the button several times more to figure out where the consumer’s break-even point is in terms of how much \(x_{2}\) is needed to balance the gain from the additional unit of \(x_{1}\). Offer 4,2.5 and then try taking away less of good 2, such as 2.7 or 2.9. Once you find the point where the amount of \(x_{2}\) taken away exactly balances the gain in \(x_{1}\) of one unit (from 3 to 4), you have located two points on a single indifference curve. If it is difficult to see the points on the chart, use the Zoom control to magnify the screen (say to 200%).
You should find that this consumer is indifferent between the bundles 3,3 and 4,2.9.
STEP Now click the button.
One hundred pairwise comparisons are made between 3,3 and a random set of alternatives. It is easy to see that the consumer can compare each and every point on the chart to the benchmark bundle of 3,3 and judge each and every point as better, worse, or the same.
STEP Click the button to display the indifference curve that goes through the benchmark point (3,3), as shown in Figure 2.1. Your version will be similar, but not exactly the same as Figure 2.1 since the 100 dots are chosen randomly.
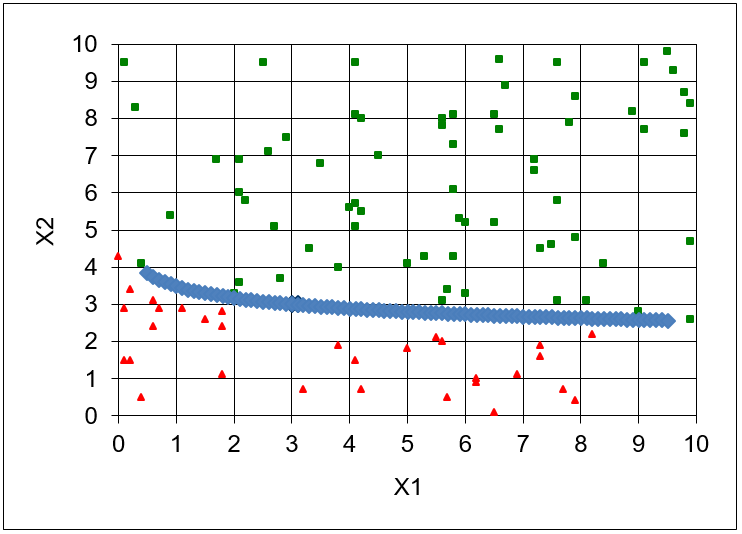
Figure 2.1: Revealing the indifference curve.
The indifference curve shows the bundles that are the same to this consumer compared to 3,3. All of the bundles for which the consumer is indifferent to the 3,3 bundle lie on the same indifference curve.
The Indifference Map
Every combination of goods has an indifference curve through it. We often display a few representative indifference curves on a chart and this is called an indifference map, as shown in Figure 2.2.
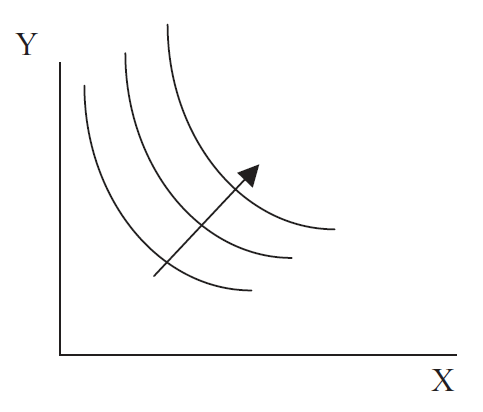Figure 2.2: An indifference map.
Any point on the curve farthest from the origin, in Figure 2.2, is preferred to any point below it, including the ones on the two lower indifference curves. The arrow indicates that satisfaction increases as you move northeast to higher indifference curves.
There are many (in fact, an infinity) of indifference curves and they are not all depicted when we draw an indifference map. We draw just a few curves. We say that the indifference map is dense, which means there is a curve through every point.
STEP Build your own indifference map by copying the Reveal sheet and clicking the button, then the button, and then the button.
This places a picture of the chart under the chart. This is an Excel drawing object, not a chart object, and it has no fill.
STEP Change the benchmark to 4,4 in cell B1 and click the button to get the indifference curve through the new benchmark point. Click the button.
This copies the chart and pastes the drawing object over the first one. Since it has no fill, it is transparent. You can separate the two pictures if you wish (click and drag), then undo the move so it is on top of the first picture.
STEP Add one more indifference curve to your map by changing the benchmark to 5,5 and clicking the button, then clicking the button.
You have created an indifference map with three representative indifference curves. Satisfaction increases as you move northeast to higher indifference curves.
Marginal Rate of Substitution
Having elicited a single indifference curve from the virtual consumer in the Excel workbook, we can define and work with a crucial concept in the Theory of Consumer Behavior: the Marginal Rate of Substitution, or MRS.
The MRS is a single number that tells us the willingness of a consumer to exchange one good for another from a given bundle. The MRS might be \(-18\) or \(-0.07\). Read carefully and work with Excel so that you learn what these numbers are telling you about the consumer’s preferences.
STEP Return to the Reveal sheet (with benchmark point 3,3) and click the button to copy and paste an image of the current indifference curve below the graph in the Reveal sheet. Now click the button to get a new virtual consumer with different preferences and then display the indifference curve for this new consumer (by clicking the button).
Notice that the indifference curve is not the same as the original one. These are two different consumers with different preferences. You can use the buttons to offer the new consumer bundles that can be compared with the 3,3 benchmark bundle, just like before.
The key idea here is that at 3,3, we can measure each consumer’s willingness to trade \(x_{2}\) in exchange for \(x_{1}\).
Initially (as shown in Figure 2.1 and in the picture you took), we saw that the consumer was indifferent between 3,3 and 4,2.9. For one more unit of \(x_{1}\) (from 3 to 4), the consumer is willing to trade 0.1 units of \(x_{2}\) (from 3 to 2.9). Then the MRS of \(x_{1}\) for \(x_{2}\) from 3,3 to 4,2.9 is measured by \(\frac{-0.1}{1}\), or \(-0.1\).
With our new virtual consumer, the MRS at 3,3 is a different number. Let’s compute it.
STEP Proceed to the MRS sheet. Click the button. Not only is the indifference curve through 3,3 displayed for this consumer, it also shows some of the bundles that lie on this indifference curve. We can use this information to compute the MRS.
You can compute the MRS at 3,3 by looking at the first bundle after 3,3. How much \(x_{2}\) is the consumer willing to give up in order to get 0.1 more of \(x_{1}\)? This ratio, \(\frac{\Delta x_2}{\Delta x_1}\), (the usual "rise over the run" definition of the slope), is the slope of the indifference curve, which is also the MRS.
The MRS also can be computed as the slope of the indifference curve at a point by using derivatives. Instead of computing \(\frac{\Delta x_2}{\Delta x_1}\) along an indifference curve from one point to another, one can find the instantaneous rate of change at 3,3. We will do this later.
The crucial concept right now is that the MRS is a number that measures the willingness of a consumer to trade one good for another at a specific point. We usually think of it in terms of giving up some of the good on the y axis to get more of the good on the x axis.
Do not fall into the trap of thinking of the MRS as applying to the entire indifference curve. In fact, the MRS is different at each point on the curve. For a typical indifference curve like in Figure 2.1, the MRS gets smaller (in absolute value) as we move down the curve (as it flattens out).
The MRS is negative because the indifference curve is sloping downwards: a decrease in \(x_{2}\) is compensated for by an increase in \(x_{1}\). We often drop the minus sign because comparing negative numbers can be confusing. For example, say one consumer has an MRS of \(-1\) at 3,3 while another has an MRS of \(-\frac{1}{3}\) at that point. It is true that \(-1\) is a smaller number than \(-\frac{1}{3}\), however, we to use the MRS to indicate the steepness of the slope. Thus, to avoid confusion, we make the comparison using the absolute value of the MRS.
Figure 2.3: Comparing MRS.
Figure 2.3 shows that the bigger in absolute value is the MRS, the more the consumer is willing to trade the good on the y axis for the good on the x axis. Thus, an MRS of \(-1\) at 3,3 means the indifference curve has a steeper slope at that point than if the MRS was \(-\frac{1}{3}\). We would say the MRS is bigger at \(-1\) than \(-\frac{1}{3}\) even though \(-1\) is a smaller number than \(-\frac{1}{3}\) because we look only at the absolute value of the MRS.
Funky Preferences and Their Indifference Curves
We can depict a wide variety of preferences with indifference maps. Here are some examples.
Example 1: Perfect Substitutes — constant slope (MRS)
If the consumer perceives two things as perfectly substitutable, it means they can get the same satisfaction by replacing one with the other.
Consider having one five-dollar bill and five one-dollar bills (as long as we are not talking about several hundred dollars worth of bills). If the consumer does not care about having $10 as a single ten-dollar bill, one five-dollar bill and five one-dollar bills, or ten one-dollar bills, then the indifference curve is a straight line as shown in Figure 2.4.
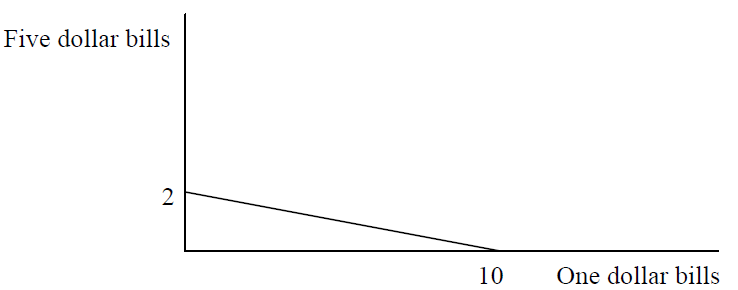Figure 2.4: Perfect substitutes.
You could argue that there is an indivisibility here and there are actually just 3 points that should not be connected by a line, but the key idea is that the indifference curve is a straight line in the case of perfect substitutes. It has a constant MRS (the slope of the line is \(-\frac{1}{5}\)), unlike a typical indifference curve where the MRS falls (in absolute value) as you move down the curve.
Example 2: Perfect Complements — L-shaped Indifference Curves
The polar opposite of perfect substitutes are perfect complements. Suppose the goods in questions have to be used in a particular way, with no room for any flexibility at all, like cars and tires. You need four tires for a car to work. With only three tires the car is worthless. Ignoring the spare, having more than four tires does not help you if you still have just one car.
Figure 2.5 illustrates the indifference map for this situation. It says that eight tires with one car gives the same satisfaction as four tires with one car. It also says that eight tires and two cars is preferred to four tires and one car (or eight tires and one car) because the middle L-shaped indifference curve (\(I_{1}\)) is farther from the origin than the lowest indifference curve (\(I_{0}\)).
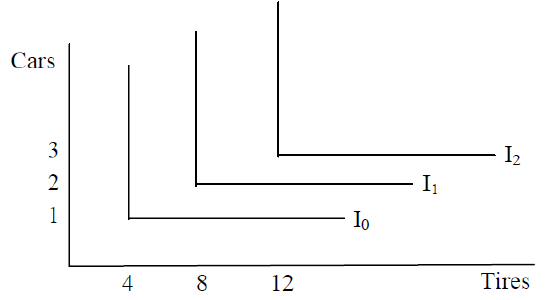Figure 2.5: Perfect complements.
Notice how the usual indifference curve lies between the two extremes of perfect substitutes (straight lines) and perfect complements (L-shaped). Thus, the typical indifference curve reflects a level of substitutability between goods that is more than perfect complements (one good cannot replace another at all), but less than perfect substitutes (one good can take the place of another with no loss of satisfaction).
Example 3: Bads
What if one of the goods is actually a bad, something that lowers satisfaction as you consume more of it, like pollution? Figure 2.6 shows the indifference map in this case.
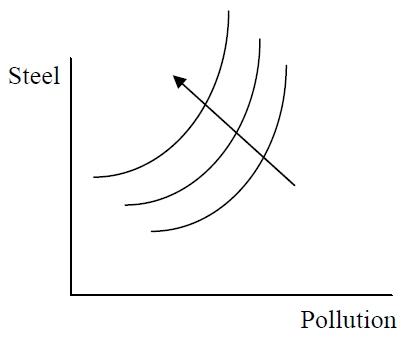Figure 2.6: Bads.
Along any one of the indifference curves, more steel and more pollution are equally satisfying because pollution is a bad that cancels out the additional good from steel. The arrow indicates that satisfaction increases by moving northwest, to higher indifference curves.
Example 4: Neutral Goods
What if the consumer thinks something is neither good nor bad? Then it is a neutral good and the indifference map looks like Figure 2.7.
The horizontal indifference curves for the neutral good on the x axis in Figure 2.7 tell you that the consumer is indifferent if offered more X. The arrow indicates that satisfaction rises as you move north (because Y is a good and having more of it increasing satisfaction).
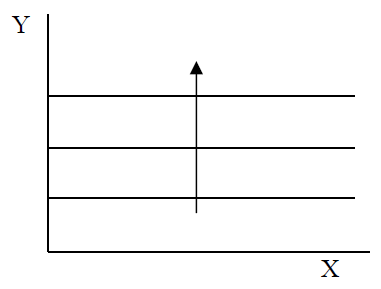Figure 2.7: X is a neutral good.
These are just a few examples of how a variety of preferences can be depicted with an indifference map. When we want to describe generic, typical preferences that produce downward sloping indifference curves, as in Figure 2.2, economists use the phrase “well-behaved preferences.”
Another technical term that is often used in economics is convexity, as in convex preferences. This means that midpoints are preferred to extremes. In Figure 2.8, there are two extreme points, A and B, which are connected by a dashed line. Any point on the dashed line, like C, can be described by the equation \(zA + (1-z)B\), where \(0 < z < 1\) controls the position of C. This equation is called a convex combination.
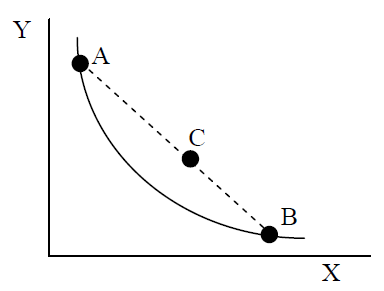Figure 2.8: Convex preferences.
If preferences are convex, then midpoints like C are strictly preferred to extreme points like A and B. Convexity is used as another way of saying that preferences are well-behaved.
An important property that arises out of well-behaved or convex preferences is that of diminishing MRS. As explained earlier, the MRS varies along an indifference curve and applies to a specific point (not to the entire curve). The MRS will start large (in absolute value) at the top left corner, like point A in Figure 2.8, and get smaller as we travel down the indifference curve to point B. This makes common sense. The consumer is readily willing to trade a lot of Y for X (so the MRS is high in absolute value) when he has a lot of Y and little X. When the amounts are reversed, such as point B, a small MRS means he is willing to give up very little Y (since he has little of it) for more X (which he has a lot of already).
Indifference Curves Reflect Preferences
Preferences, a consumer’s likes and dislikes, can be elicited or revealed by asking the consumer to pick between pairs of bundles. The indifference curve is that set of bundles that the consumer finds equally satisfying.
The MRS is a single number that measures the willingness of the consumer to exchange one good for another at a particular point. If the MRS is high (in absolute value), the indifference curve is steep at that point and the consumer is willing trade a lot of Y for a little more X.
Standard, well-behaved preferences yield a set of smooth arcs (like Figure 2.2), but there are many other shapes that depict preferences for different kinds of goods and the relationship between goods.
Exercises
What is the MRS at any point if X is a neutral good? Explain why.
If the good on the y axis was a neutral good and the other good was a regular good, then what would the indifference map look like. Use Word’s Drawing Tools to draw a graph of this situation.
If preferences are well-behaved, then indifference curves cannot cross. Use Figure 2.9 to help you construct an explanation for why this claim must be true. Note that point C has more X and Y than point A, thus, by insatiability, C must be preferred to A. The key to defending the claim lies in the assumption of transitivity.
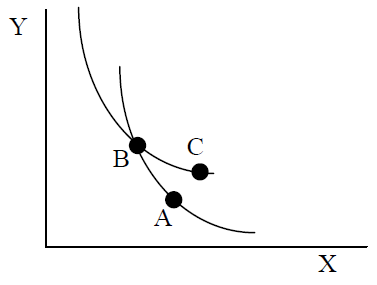Figure 2.9: An impossible indifference map.
Suppose we measure consumer A’s and B’s MRS at the same point and find that \(MRS_{A} = - 6\) and the \(MRS_{B} = - 2\). What can we say about the preferences of A and B at this point?
References
The epigraph is from page 26 of C. E. Ferguson’s Microeconomic Theory (revised edition, 1969), a popular micro text in the 1960s and 1970s. In the preface Ferguson wrote, “This is a textbook; its content is taken from the public domain of economic literature. Conventional topics are treated in conventional ways; and there is no real innovation.” Perhaps, but Ferguson adopted a much more mathematical presentation and added content, including general equilibrium theory, that made his book different and important.
button. For bundle B, enter 4, then a comma (,), then a 3, then click OK.
button.
button to display the indifference curve that goes through the benchmark point (3,3), as shown in Figure 2.1. Your version will be similar, but not exactly the same as Figure 2.1 since the 100 dots are chosen randomly.
Figure 2.2: An indifference map.
button, then the 
button, and then the 
button.
button to get the indifference curve through the new benchmark point. Click the 
button.
button, then clicking the 
button to copy and paste an image of the current indifference curve below the graph in the Reveal sheet. Now click the 
button to get a new virtual consumer with different preferences and then display the indifference curve for this new consumer (by clicking the 
button).
button. Not only is the indifference curve through 3,3 displayed for this consumer, it also shows some of the bundles that lie on this indifference curve. We can use this information to compute the MRS.
Figure 2.3: Comparing MRS.
Figure 2.4: Perfect substitutes.
Figure 2.5: Perfect complements.
Figure 2.6: Bads.
Figure 2.7: X is a neutral good.
Figure 2.8: Convex preferences.
Figure 2.9: An impossible indifference map.